categories
recent posts
tags
Invitation for the Photonics West 2023 WTS would attend the USA Photonics West 2023 at booth #5533 Jan 31th to Feb 2nd 2023 Welco...
China International Optoelectronic Expo CIOE 2021 Time: Sep 16th-18th 2021 Add: ShenZhen World Exhibition & Convention Center Booth Number: #5A75 ...
WORKING TOGETHER, FIGHTING WITH COVID 2019 Dear Customer, Partners and Colleagues, In 2020, we spent the longest and most memorable Spring Festival in...
Happy 2020 Chinese Rat Year At 6:00 pm on January 10, 2020, the Fuzhou WTS Photonics 2019 End of the Year Party was kicked off at the Hyat...
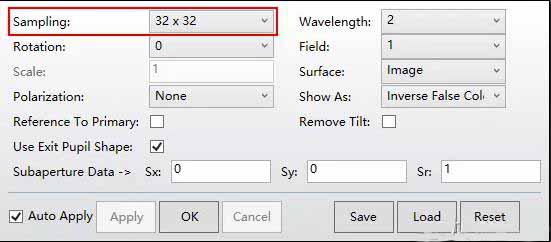
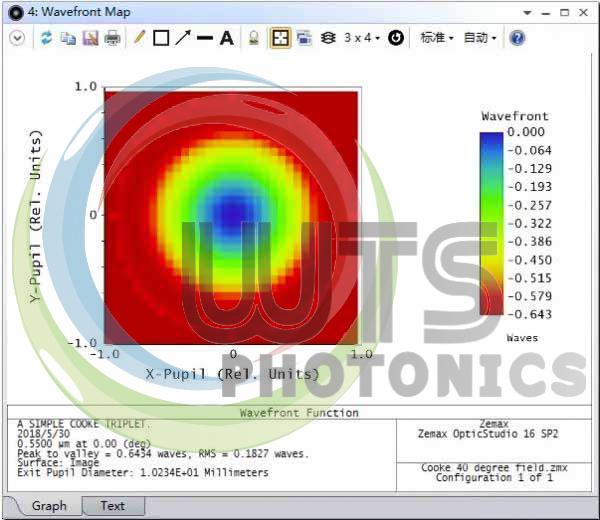
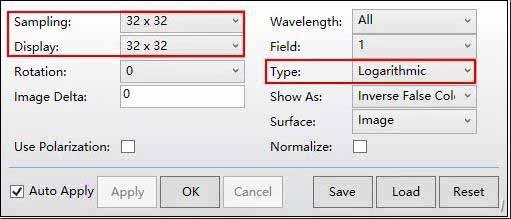
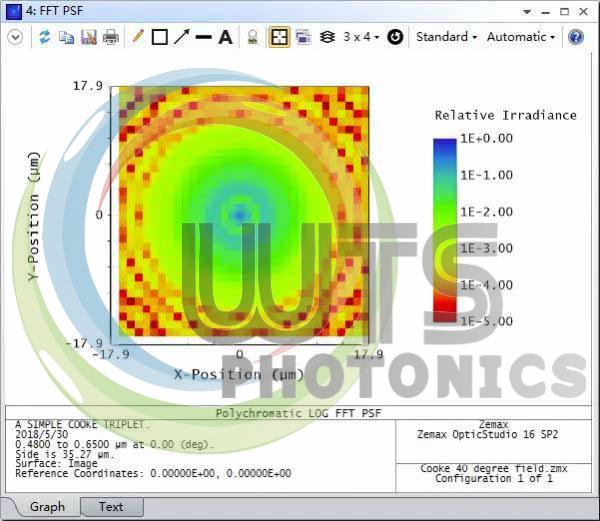
friendly links :
copyright © 2024 WTS PHOTONICS CO., LTD.all rights reserved. powered by dyyseo.com .
wts photonics